形式三角矩阵环上的Gorenstein FI-内射模
Gorenstein FI-Injective Modules Over Formal Triangular Matrix Rings
DOI:10.12677/PM.2023.1312390,PDF,下载: 113浏览: 174
作者:赵为东：西北师范大学数学与统计学院，甘肃 兰州
关键词:形式三角矩阵环；FI-内射模；Gorensteimn FI-内射模；Formal Triangular Matrix Ring；FI-Injective Module；Gorenstein FI-Injective Module
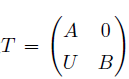
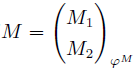
摘要:本文研究了形式三角矩阵环上的Gorenstein FI- 内射模。设 是形式三角矩阵环，其中A，B 是环，U 是(B,A)-双模。在一定条件下证明了若 是Gorenstein FI-内射左T-模，则M 2是Gorenstein FI-内射左B-模，ker φM ~是Gorenstein FI- 内射左A- 模，并且 φM ~是满同态。
Abstract:Let be a formal triangular matrix ring, where A and B are rings and U is (B,A)-bimodule. This article proves under certain conditions that if is a Gorenstein FI-injective left T-modules, then M2 is a Gorenstein FI-injective left B-modules, ker φM ~is a Gorenstein FI-injective left A-module, and φM ~is an epimorphism.
文章引用：赵为东. 形式三角矩阵环上的Gorenstein FI-内射模[J]. 理论数学, 2023, 13(12): 3771-3779. https://doi.org/10.12677/PM.2023.1312390

参考文献

[1] Auslander, M. and Brideg, M. (1969) Stable Module Theory. In: Memoirs of the American Mathematical Society, Vol. 94, American Mathematical Society, Providence, RI.
https://doi.org/10.1090/memo/0094
[2] Enochs, E.E. and Jenda, O.M.G. (1995) Gorenstein Injective and Projective Modules. Mathe- matische Zeitschrift, 220, 611-633.
https://doi.org/10.1515/9783110215212
[3] Zhang, P. (2013) Gorenstein-Projective Modules and Symmetric Recollements. Journal of Al- gebra, 388, 65-80.
https://doi.org/10.1016/j.jalgebra.2013.05.008
[4] Enochs, E.E., Cortes-Izurdiaga, M. and Torrecillas, B. (2014) Gorenstein Conditions over Triangular Matrix Rings. Journal of Pure and Applied Algebra, 218, 1544-1554.
https://doi.org/10.1016/j.jpaa.2013.12.006
[5] Mao, L. and Ding, N. (2007) FI-Injective and FI-Flat Modules. Journal of Algebra, 309, 367- 385.
https://doi.org/10.1016/j.jalgebra.2006.10.019
[6] Mao, L., Ding, N. and Zelmanov, E. (2008) Gorenstein FP-Injective and Gorenstein Flat Modules. Journal of Algebra and Its Applications, 7, 491-506.
[7] 陈东,胡葵.关于Gorenstein FI-内射模[J]. 西北师范大学学报(自然科学版), 2019,55(3): 9-13.
https://doi.org/10.16783/j.cnki.nwnuz.2019.03.03
[8] Mao, L.X. (2020) Duality Pairs and FP-Injective Modules over Formal Triangular Matrix Rings. Communications in Algebra, 48, 5296-5310.
https://doi.org/10.1080/00927872.2020.1786837
[9] 杨银银,张翠萍.形式三角矩阵环上的Gorenstein FP-内射模[J].山东大学学报(理学版),2022, 57(20): 38-44.
[10] Haghany, A. and Varadarajan, K. (1999) Study of Formal Triangular Matrix Rings. Commu- nications in Algebra, 27, 5507-5525.
https://doi.org/10.1080/00927879908826770
[11] Haghany, A. and Varadarajan, K. (2000) Study of Modules over Formal Triangular Matrix Rings. Journal of Pure and Applied Algebra, 147, 41-58.
https://doi.org/10.1016/S0022-4049(98)00129-7
[12] 佟文廷.同调代数引论[M].北京: 高等教育出版社，1998.

为你推荐



Baidu
map